贝叶斯定理

1 .Bayes Theorem

2 .Motivating Example: Drug Tests A drug test gives a false positive 2% of the time (that is, 2% of those who test positive actually are not drug users) And the same test gives a false negative 1% of the time (that is, 1% of those who test negative actually are drug users) If Joe tests positive, what are the odds Joe is a drug user? Insufficient information! Suppose we know that 1% of the population uses the drug?

3 .Setting Up the Drug Test Problem Let T be the set of people who test positive Let D be the set of drug users These are events, and Pr(T|D ) is the probability that a drug user tests positive Pr(T|D ) = .99 because the false negative rate is 1%, that is, 99% of drug users test positive, 1% test negative We want to know: What is Pr(D|T )? This is a very different question: What is the probability you are a drug user, given that you test positive?

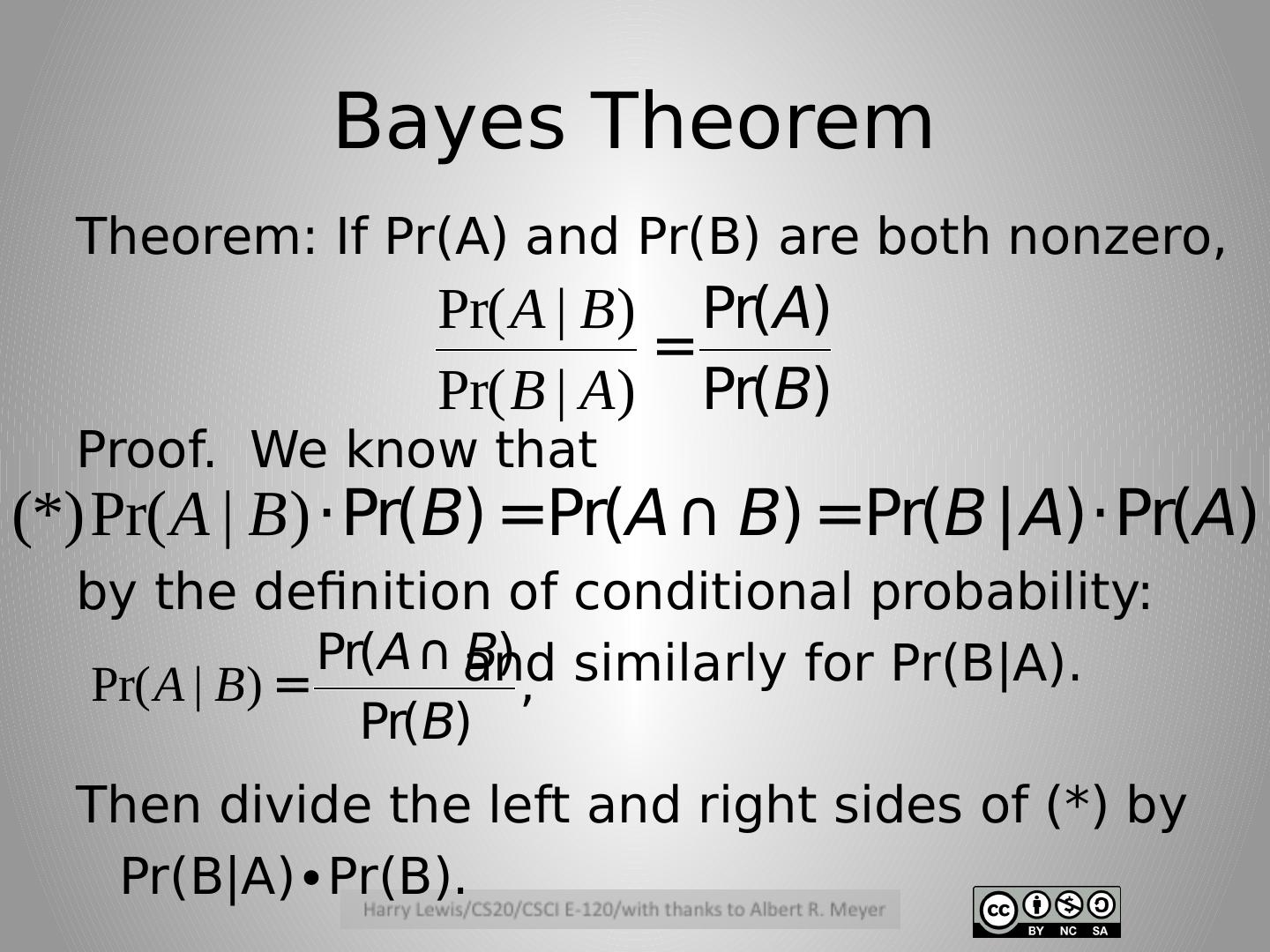
4 .Bayes Theorem Theorem: If Pr(A ) and Pr(B ) are both nonzero, Proof. We know that by the definition of conditional probability: and similarly for Pr(B|A ). Then divide the left and right sides of (*) by Pr (B|A)∙Pr(B ).

5 .Bayes , v . 2 This enables us to calculate Pr(A|B ) using only the absolute probability Pr(A ) and the conditional probabilities Pr(B|A ) and Pr(B|¬A ). Proof. We know that Now multiply by Pr(B|A ) and rewrite Pr(B ) using the law of total probability.

6 .Drug Test again Suppose that a drug test has 2% false positives (that is, 2% of the people who test positive are not drug users ) 1% false negatives (1% of those who test negative are drug users). Suppose 1% of the population uses drugs. If you test positive, what are the odds you are actually a drug user?

7 .Drug test, cont’d Let D = “Uses drugs” Let T = “Tests positive” What is Pr(D|T )?

8 .If you fail the drug test, there is only one chance in three you are actually a drug user! How can this be? Think about it. Out of 1000 people there are 10 drug users and 990 non-users. Of those 990, 2% or almost 20 test positive. Almost all of the 10 users also test positive. So there are 2 non-users for every user, among those who test positive!

9 .FINIS