展开查看详情
1 . Lecture #4
ANNOUNCEMENTS
• Prof. King will not hold office hours this week, but will hold
an extra office hour next Mo (2/3) from 11AM-12:30PM
• Quiz #1 will be given at the beginning of class on Th 2/6
– covers material in Chapters 1 & 2 (HW#1 & HW#2)
– closed book; one page of notes allowed
OUTLINE
– Drift (Chapter 3.1)
» carrier motion
» mobility
» resistivity
Spring 2003 EE130 Lecture 4, Slide 1
Nondegenerately Doped Semiconductor
• Recall that the expressions for n and p were derived using
the Boltzmann approximation, i.e. we assumed
Ev + 3kT ≤ EF ≤ Ec − 3kT
The semiconductor is said to be nondegenerately doped in this case.
Spring 2003 EE130 Lecture 4, Slide 2
1
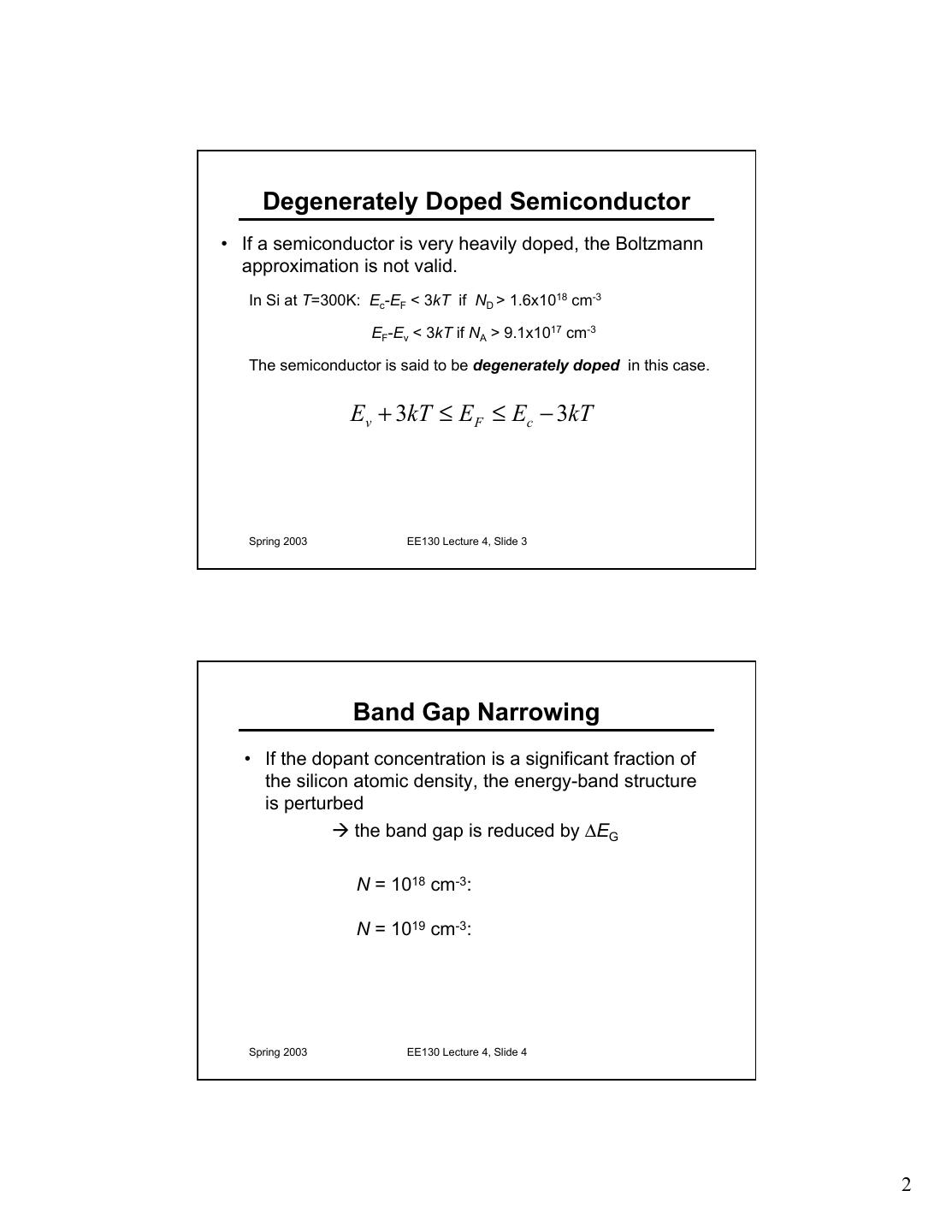
2 . Degenerately Doped Semiconductor
• If a semiconductor is very heavily doped, the Boltzmann
approximation is not valid.
In Si at T=300K: Ec-EF < 3kT if ND > 1.6x1018 cm-3
EF-Ev < 3kT if NA > 9.1x1017 cm-3
The semiconductor is said to be degenerately doped in this case.
Ev + 3kT ≤ EF ≤ Ec − 3kT
Spring 2003 EE130 Lecture 4, Slide 3
Band Gap Narrowing
• If the dopant concentration is a significant fraction of
the silicon atomic density, the energy-band structure
is perturbed
Æ the band gap is reduced by ∆EG
N = 1018 cm-3:
N = 1019 cm-3:
Spring 2003 EE130 Lecture 4, Slide 4
2
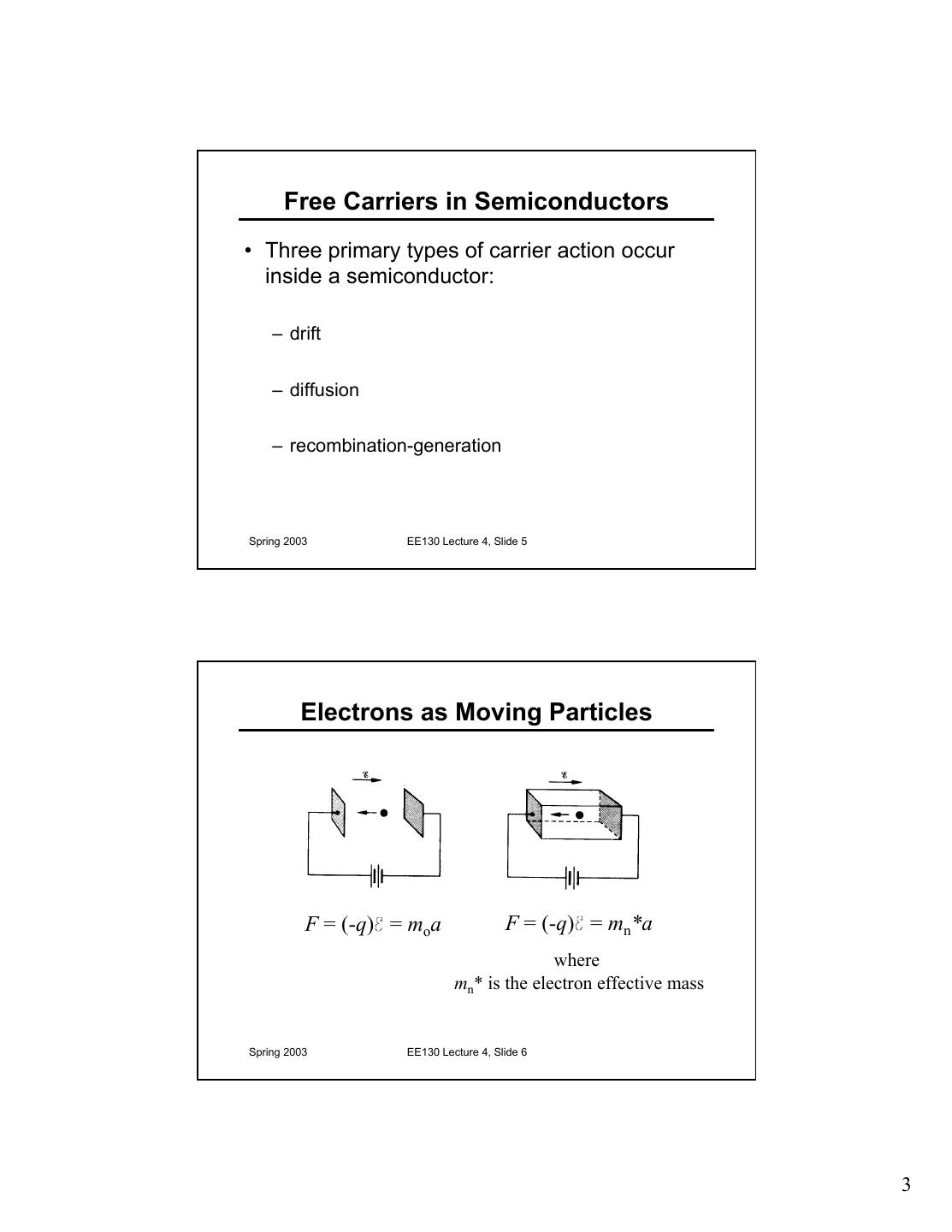
3 . Free Carriers in Semiconductors
• Three primary types of carrier action occur
inside a semiconductor:
– drift
– diffusion
– recombination-generation
Spring 2003 EE130 Lecture 4, Slide 5
Electrons as Moving Particles
F = (-q) = moa F = (-q) = mn*a
where
mn* is the electron effective mass
Spring 2003 EE130 Lecture 4, Slide 6
3
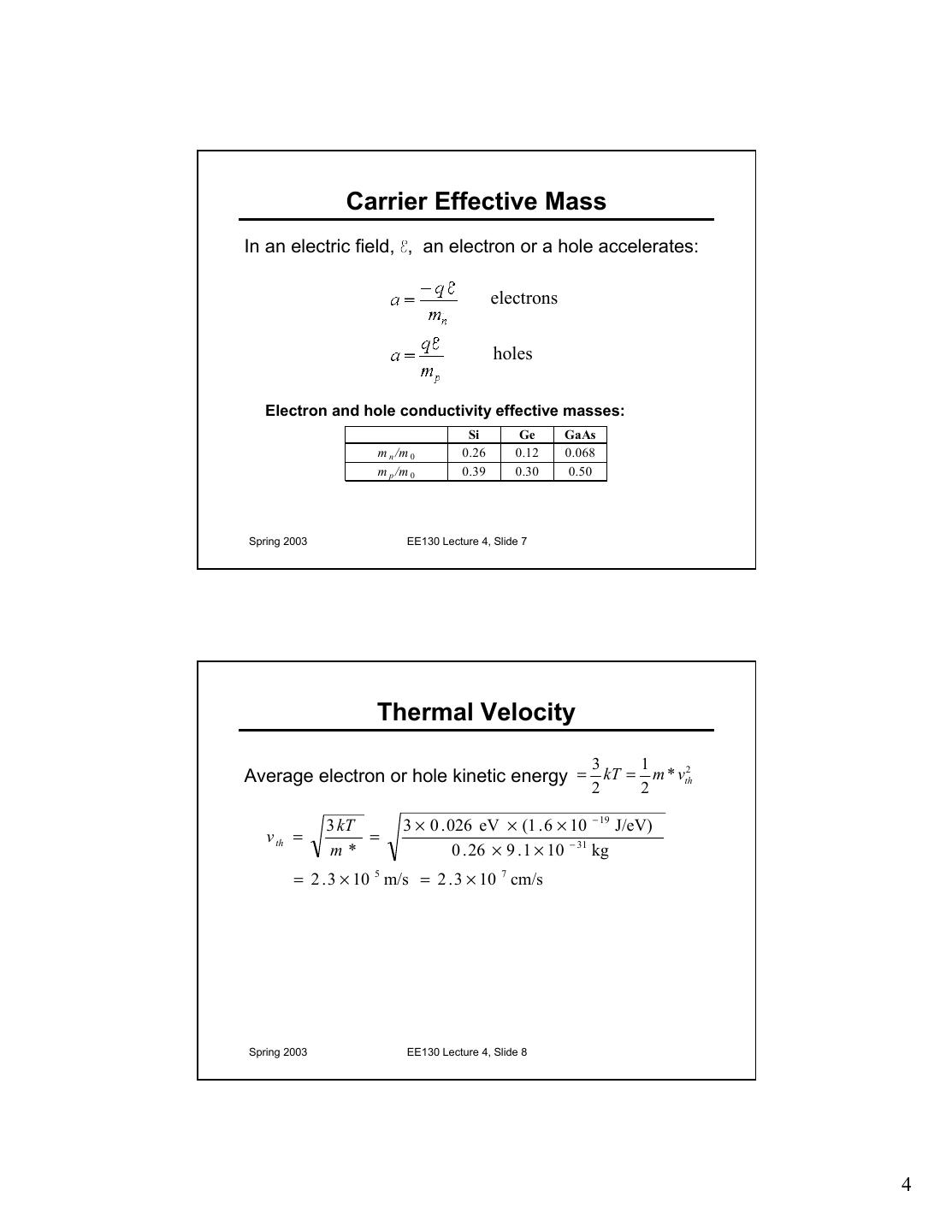
4 . Carrier Effective Mass
In an electric field, , an electron or a hole accelerates:
electrons
holes
Electron and hole conductivity effective masses:
Si Ge GaAs
m n /m 0 0.26 0.12 0.068
m p /m 0 0.39 0.30 0.50
Spring 2003 EE130 Lecture 4, Slide 7
Thermal Velocity
3 1
Average electron or hole kinetic energy = kT = m * vth
2
2 2
3 kT 3 × 0 . 026 eV × (1 . 6 × 10 − 19 J/eV)
v th = =
m* 0 . 26 × 9 . 1 × 10 − 31 kg
= 2 . 3 × 10 5 m/s = 2 . 3 × 10 7 cm/s
Spring 2003 EE130 Lecture 4, Slide 8
4
5 . Carrier Scattering
• Mobile electrons and atoms in the Si lattice are
always in random thermal motion.
– Electrons make frequent collisions with the vibrating atoms
• “lattice scattering” or “phonon scattering”
– increases with increasing temperature
– Average velocity of thermal motion for electrons: ~107 cm/s @ 300K
• Other scattering mechanisms:
– deflection by ionized impurity atoms
– deflection due to Coulombic force between carriers
• “carrier-carrier scattering”
• only significant at high carrier concentrations
• The net current in any direction is zero, if no electric
field is applied. 2 3
1
4 electron
Spring 2003 EE130 Lecture 4, Slide 9
5
Carrier Drift
• When an electric field (e.g. due to an externally applied
voltage) is applied to a semiconductor, mobile charge-
carriers will be accelerated by the electrostatic force. This
force superimposes on the random motion of electrons:
2
3 1
4 electron
5
• Electrons drift in the direction opposite to the electric field
Æ current flows
Because of scattering, electrons in a semiconductor do not achieve
constant acceleration. However, they can be viewed as quasi-classical
particles moving at a constant average drift velocity vd
Spring 2003 EE130 Lecture 4, Slide 10
5
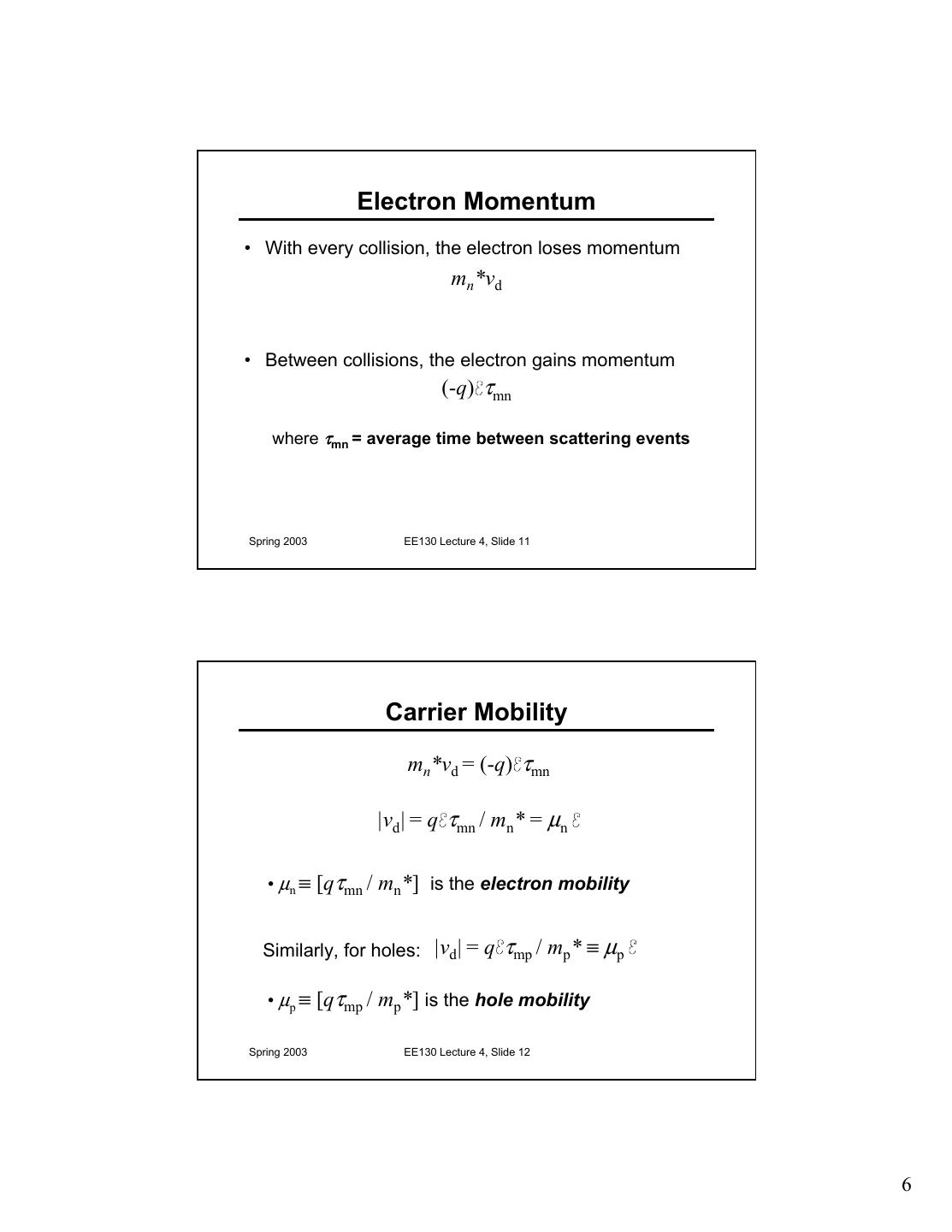
6 . Electron Momentum
• With every collision, the electron loses momentum
mn*vd
• Between collisions, the electron gains momentum
(-q) τmn
where τmn = average time between scattering events
Spring 2003 EE130 Lecture 4, Slide 11
Carrier Mobility
mn*vd = (-q) τmn
|vd| = q τmn / mn* = µn
• µn ≡ [qτmn / mn*] is the electron mobility
Similarly, for holes: |vd| = q τmp / mp* ≡ µp
• µp ≡ [qτmp / mp*] is the hole mobility
Spring 2003 EE130 Lecture 4, Slide 12
6
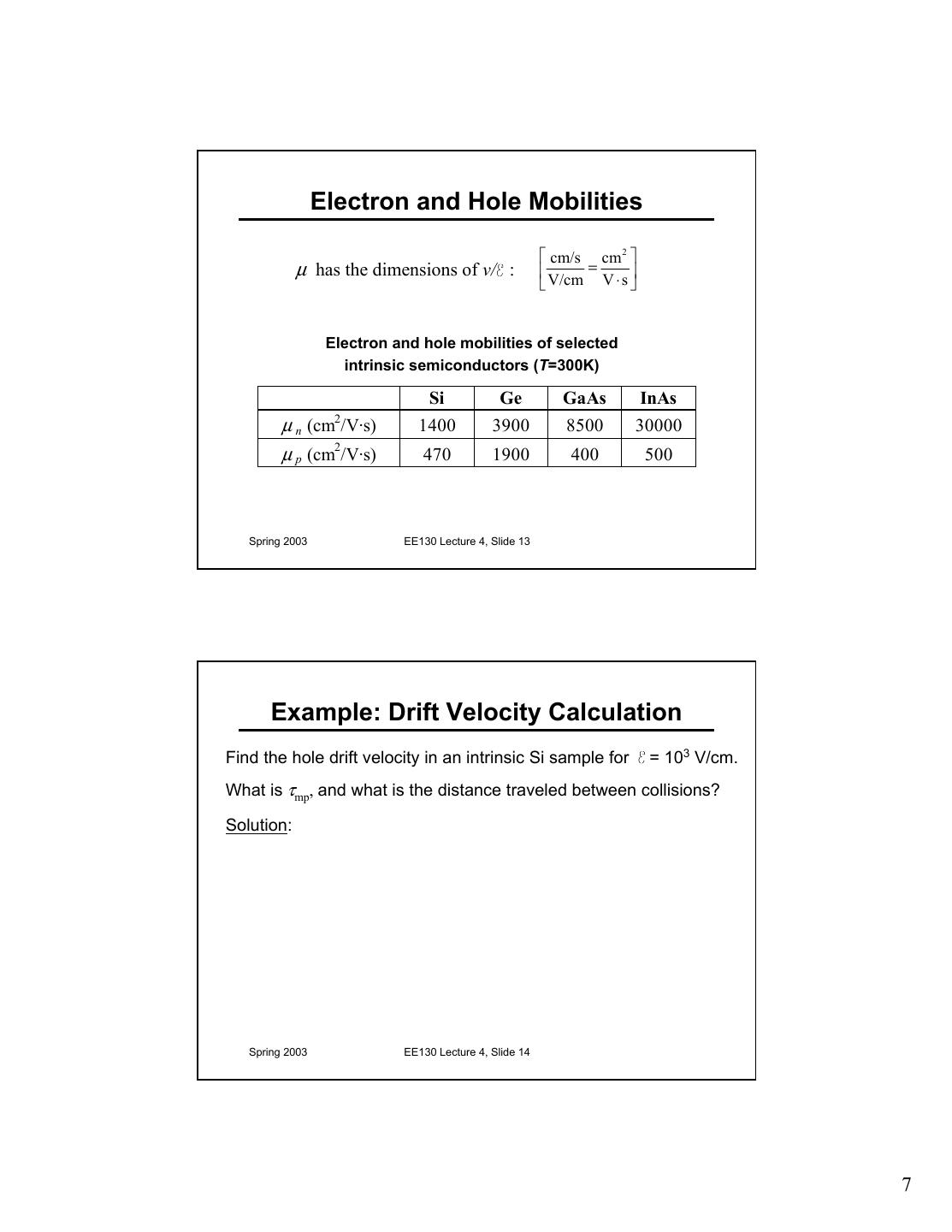
7 . Electron and Hole Mobilities
cm/s cm 2
µ has the dimensions of v/ : =
V/cm V ⋅ s
Electron and hole mobilities of selected
intrinsic semiconductors (T=300K)
Si Ge GaAs InAs
2
µ n (cm /V·s) 1400 3900 8500 30000
µ p (cm2/V·s) 470 1900 400 500
Spring 2003 EE130 Lecture 4, Slide 13
Example: Drift Velocity Calculation
Find the hole drift velocity in an intrinsic Si sample for = 103 V/cm.
What is τmp, and what is the distance traveled between collisions?
Solution:
Spring 2003 EE130 Lecture 4, Slide 14
7
8 . Mobility Dependence on Doping
1600
1400 1 1 1
= +
1200
τ τ phonon τ impurity
E lectrons
1 1 1
= +
Mobility (cm V s )
-1
1000
µ µ phonon µ impurity
-1
800
2
600
400
H o les
200
0
1E 14 1E 15 1E 16 1E 17 1E 18 1E 19 1E 20
-3
Total Doping
T otal Concentration
Im p urity C on ce nra tio nN(a
A + ND (cm )
to m s cm -3)
Spring 2003 EE130 Lecture 4, Slide 15
Drift Current
vd t A = volume from which all holes cross plane in time t
p vd t A = # of holes crossing plane in time t
q p vd t A = charge crossing plane in time t
q p vd A = charge crossing plane per unit time = hole current
Î Hole current per unit area J = q p vd
Spring 2003 EE130 Lecture 4, Slide 16
8
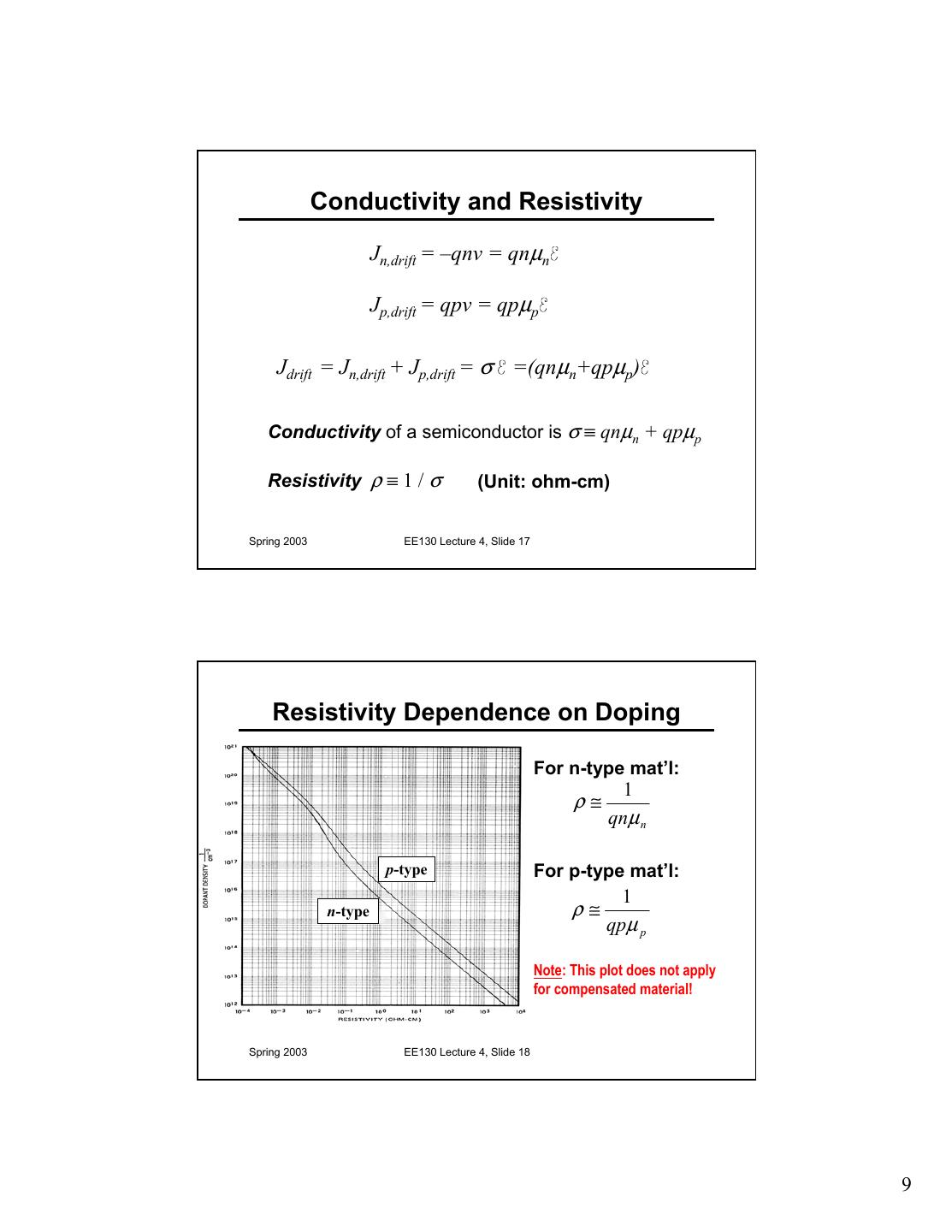
9 . Conductivity and Resistivity
Jn,drift = –qnv = qnµn
Jp,drift = qpv = qpµp
Jdrift = Jn,drift + Jp,drift = σ =(qnµn+qpµp)
Conductivity of a semiconductor is σ ≡ qnµn + qpµp
Resistivity ρ ≡ 1 / σ (Unit: ohm-cm)
Spring 2003 EE130 Lecture 4, Slide 17
Resistivity Dependence on Doping
For n-type mat’l:
1
ρ≅
qnµ n
p-type For p-type mat’l:
1
n-type ρ≅
qpµ p
Note: This plot does not apply
for compensated material!
Spring 2003 EE130 Lecture 4, Slide 18
9
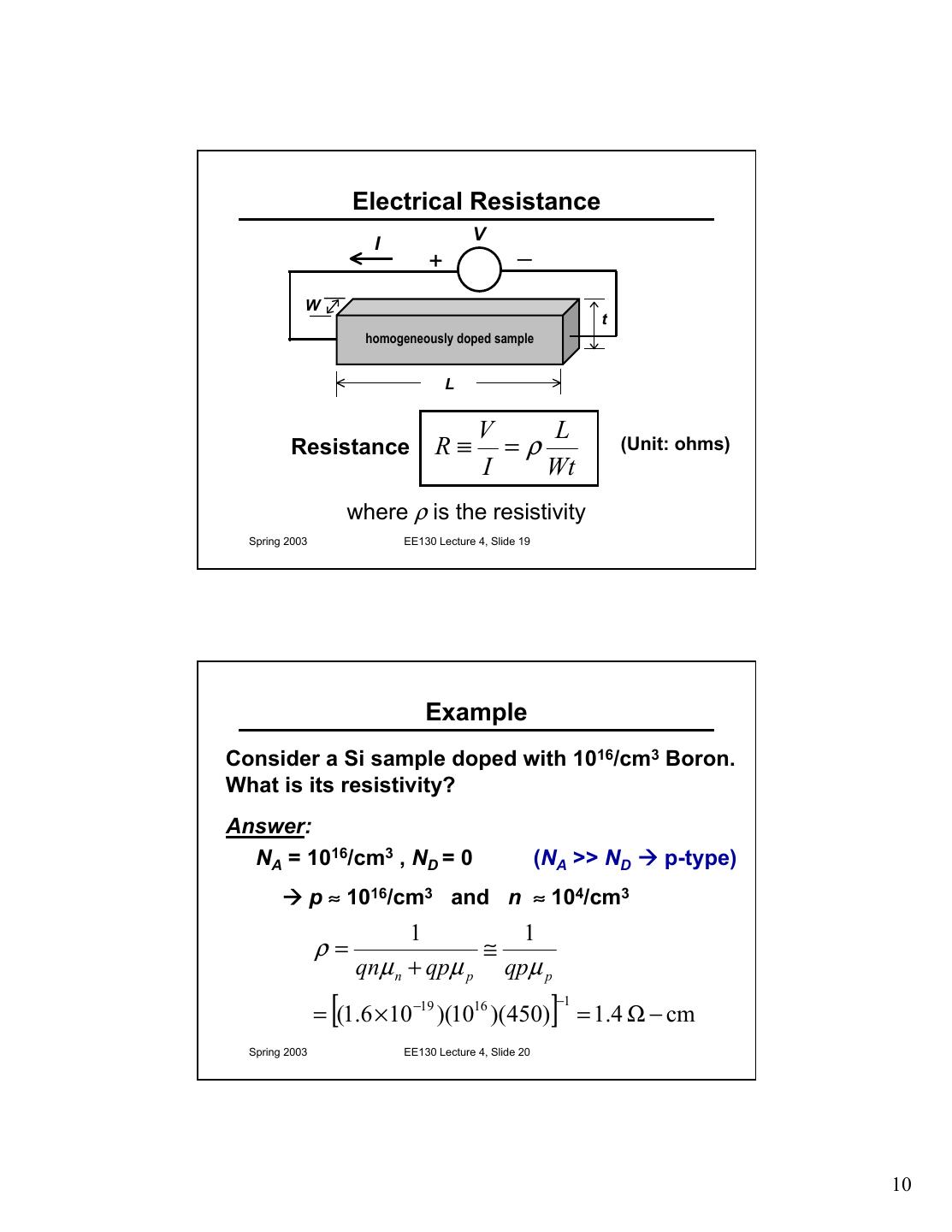
10 . Electrical Resistance
V
I _
+
W
t
homogeneously doped sample
L
V L
Resistance R≡ =ρ (Unit: ohms)
I Wt
where ρ is the resistivity
Spring 2003 EE130 Lecture 4, Slide 19
Example
Consider a Si sample doped with 1016/cm3 Boron.
What is its resistivity?
Answer:
NA = 1016/cm3 , ND = 0 (NA >> ND Æ p-type)
Æ p ≈ 1016/cm3 and n ≈ 104/cm3
1 1
ρ= ≅
qnµ n + qpµ p qpµ p
[
= (1.6 × 10 −19 )(1016 )(450) ]−1
= 1.4 Ω − cm
Spring 2003 EE130 Lecture 4, Slide 20
10
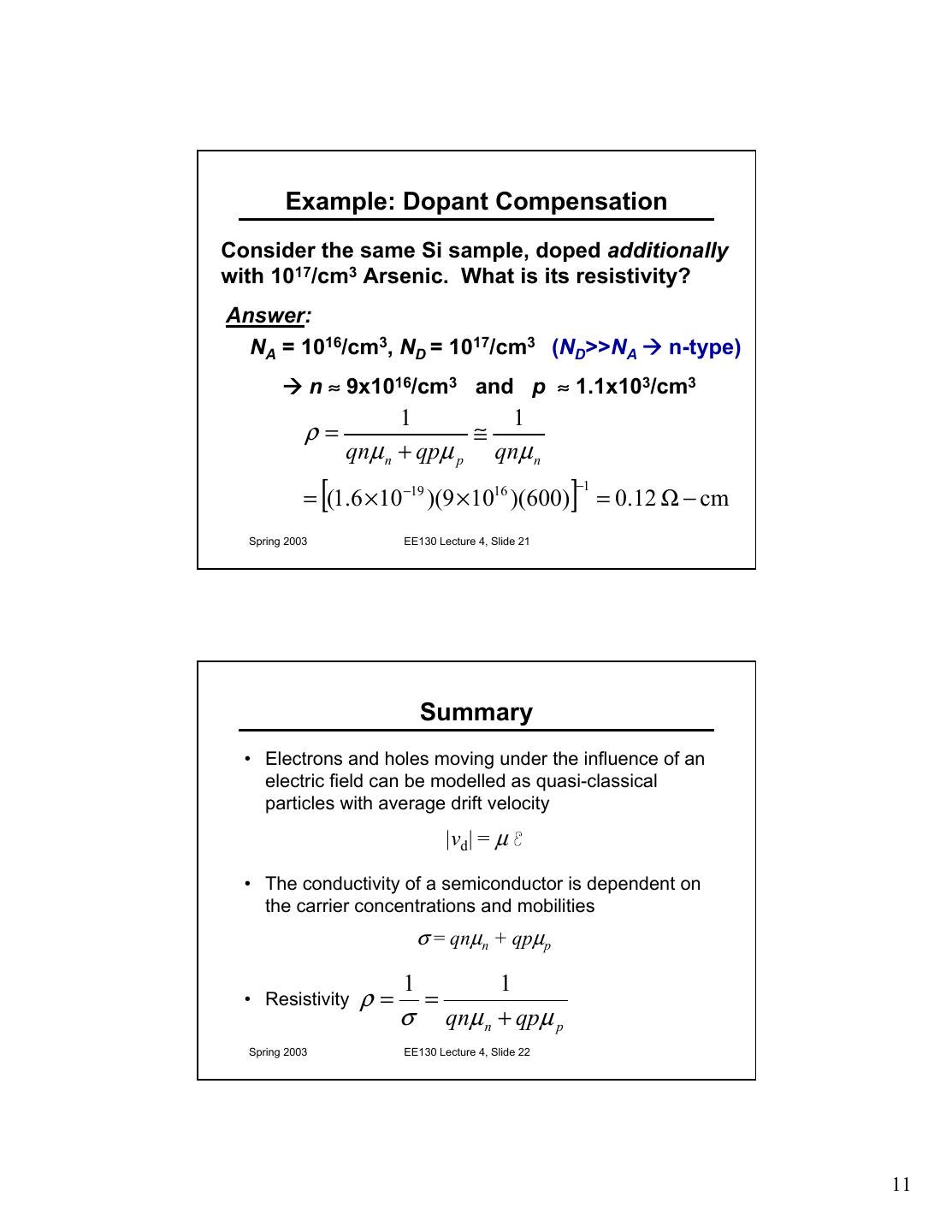
11 . Example: Dopant Compensation
Consider the same Si sample, doped additionally
with 1017/cm3 Arsenic. What is its resistivity?
Answer:
NA = 1016/cm3, ND = 1017/cm3 (ND>>NA Æ n-type)
Æ n ≈ 9x1016/cm3 and p ≈ 1.1x103/cm3
1 1
ρ= ≅
qnµ n + qpµ p qnµ n
[
= (1.6 ×10 −19 )(9 × 1016 )(600) ]
−1
= 0.12 Ω − cm
Spring 2003 EE130 Lecture 4, Slide 21
Summary
• Electrons and holes moving under the influence of an
electric field can be modelled as quasi-classical
particles with average drift velocity
|vd| = µ
• The conductivity of a semiconductor is dependent on
the carrier concentrations and mobilities
σ = qnµn + qpµp
1 1
• Resistivity ρ= =
σ qnµ n + qpµ p
Spring 2003 EE130 Lecture 4, Slide 22
11
热门城市
