展开查看详情
1 .Conditional Probability
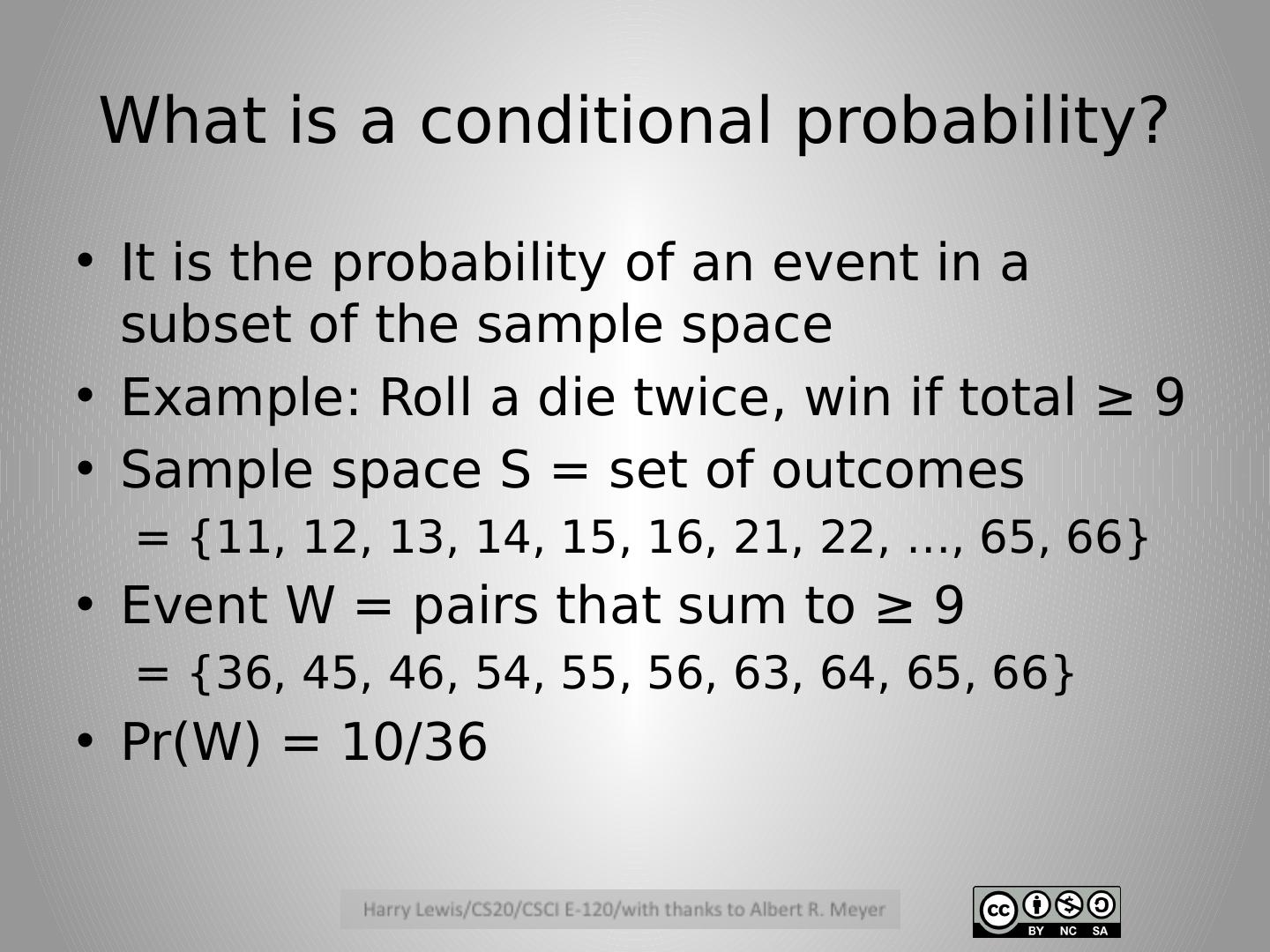
2 .What is a conditional probability? It is the probability of an event in a subset of the sample space Example: Roll a die twice, win if total ≥ 9 Sample space S = set of outcomes = {11, 12, 13, 14, 15, 16, 21, 22, …, 65, 66} Event W = pairs that sum to ≥ 9 = {36, 45, 46, 54, 55, 56, 63, 64, 65, 66} Pr (W ) = 10/36
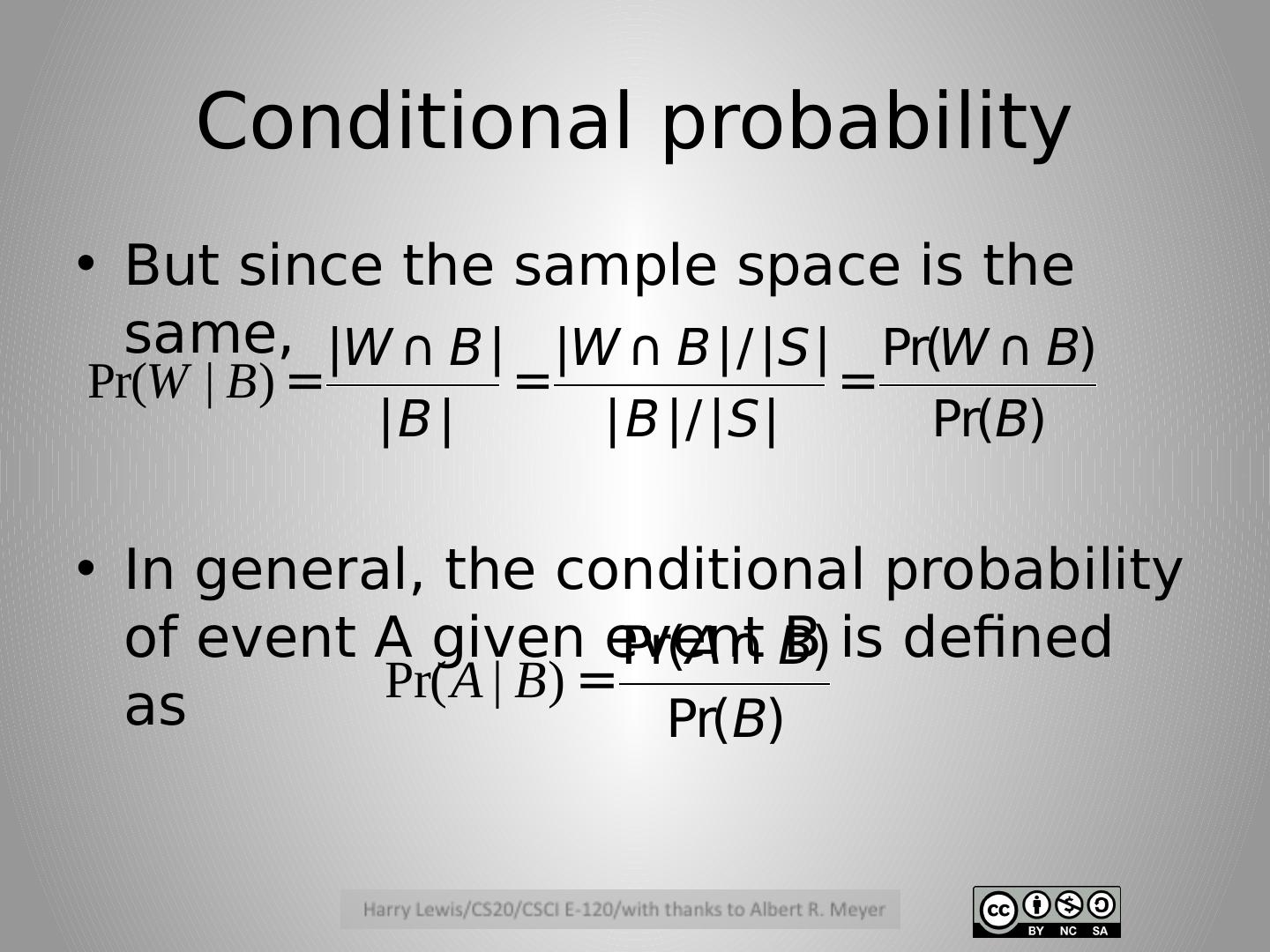
3 .What is a conditional probability? Now suppose we know that the first roll is 4 or 5 . What is now the probability that the sum of the two rolls will be ≥ 9? Let B = first roll is 4 or 5 = {41, 42, …, 46, 51 , 52, …, 56} Event W∩ B = {45, 46, 54 , 55, 56} Pr (W | B) = |W∩ B|/|B| = 5/12 “Probability of W given B”
4 .Conditional probability But since the sample space is the same, In general, the conditional probability of event A given event B is defined as
5 .What is the difference between Pr(A|B ) and Pr(B|A )? Pr(A|B ) is the proportion of B that is also within A, that is, Pr(A|B ) is |A∩B| as a proportion of |B| Pr(A|B ) is close to 1 but Pr(B|A ) is close to 0 A B A∩B
6 .CS20 This class has 42 students, 13 freshmen, 17 women, and 5 women freshmen So if a student is selected at random, Pr(Freshman ) = 13/42, Pr(Woman ) = 17/42 Pr(Woman freshman) = 5/42. If a random selection chooses a woman, what is the probability she is a freshman? Simple way: #women freshmen/#women = 5/17 Using probability:
7 .Conditional Probability and Independence Fact: A and B are independent events iff Pr( A|B ) = Pr( A ). That is, knowing whether B is the case gives no information that would help determine the probability of A. Proof: A and B independent iff Pr(A)∙Pr(B ) = Pr(A∩B ) Pr(A∩B ) = Pr(A|B)∙Pr(B ) So as long as Pr(B ) is nonzero, Pr(A)∙Pr(B ) = Pr(A|B)∙Pr(B ) iff Pr(A ) = Pr(A|B )
8 .Total Probability Suppose (hypothetically!): Rick Santorum has a 5% probability of getting enough delegates to become the Republican nominee, unless the voting goes beyond the first ballot and there is a brokered convention In a brokered convention, Santorum has a 65% probability of winning the nomination There is a 7% probability of a brokered convention (cf. Intrade.com ) What is the probability that Santorum will be the Republican nominee?
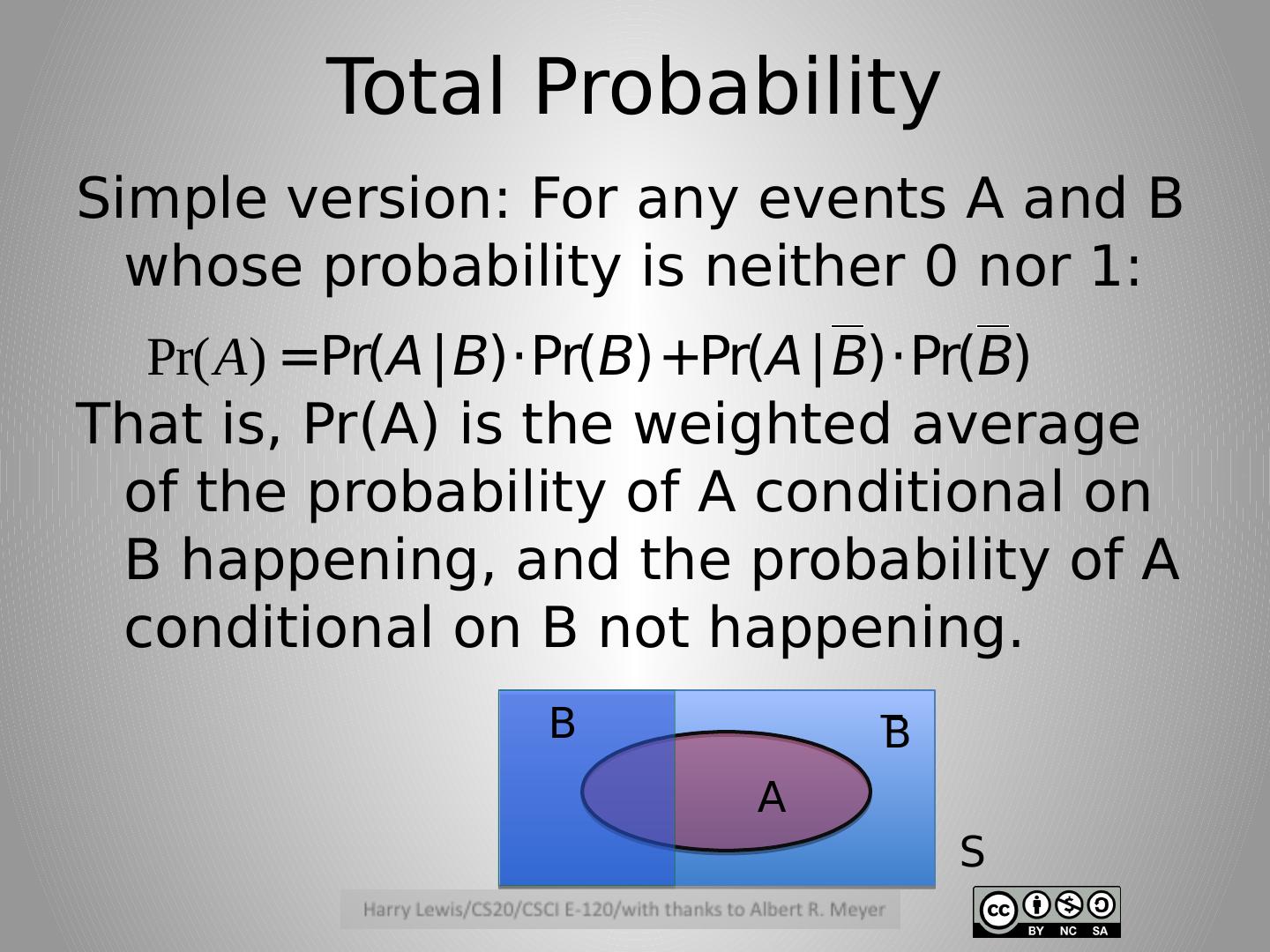
9 .Total Probability Simple version: For any events A and B whose probability is neither 0 nor 1: That is, Pr(A ) is the weighted average of the probability of A conditional on B happening, and the probability of A conditional on B not happening. B B _ A S
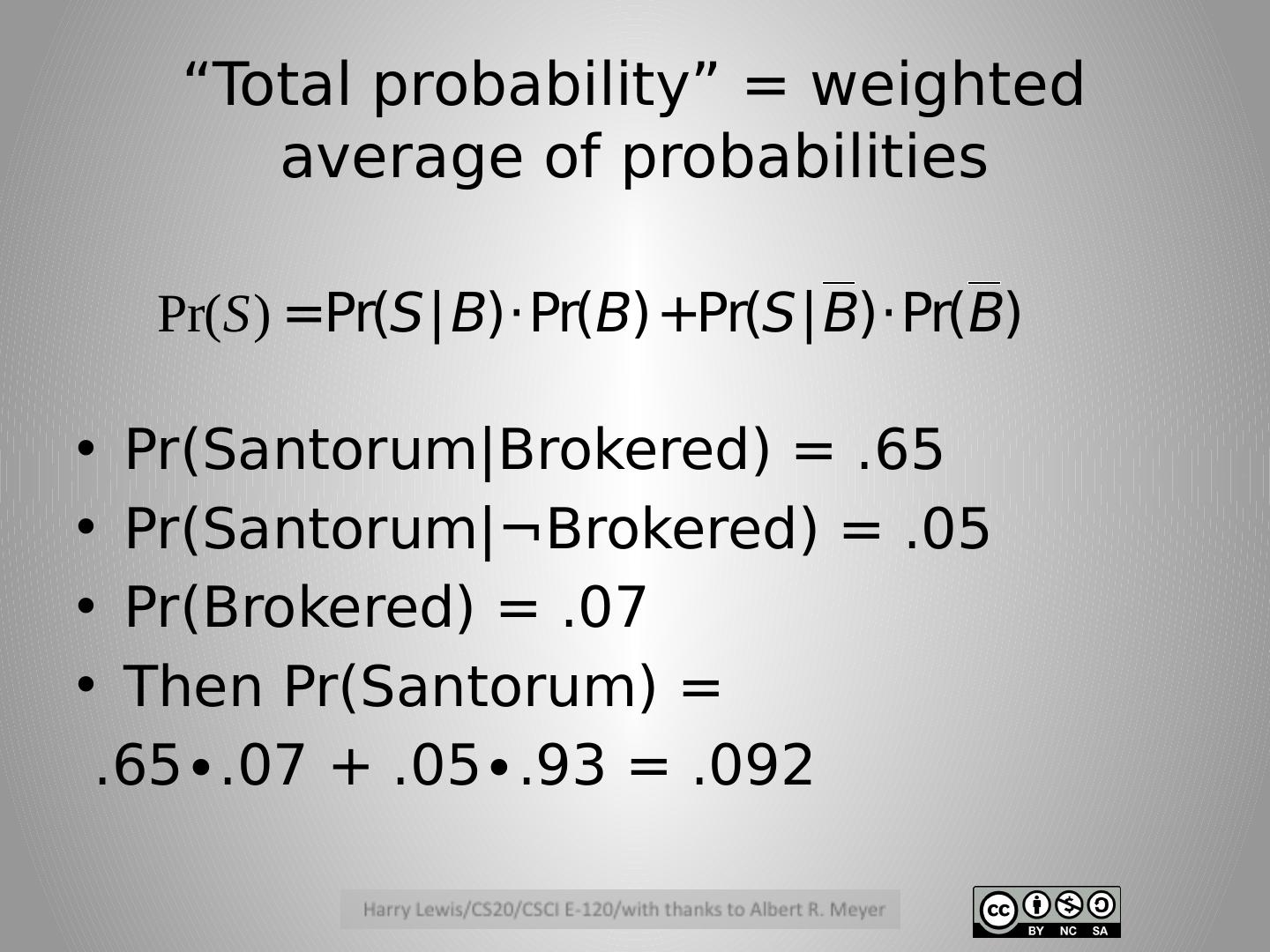
10 .“ Total probability” = weighted average of probabilities Pr(Santorum|Brokered ) = .65 Pr(Santorum|¬Brokered ) = .05 Pr(Brokered ) = . 07 Then Pr(Santorum ) = .65∙ . 07 + .05∙ . 93 = . 092
热门城市
